
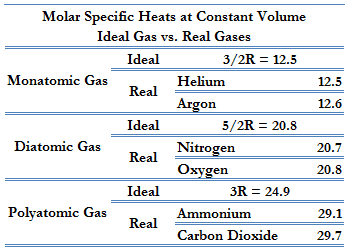
Paraffin for example has very large molecules and thus a high heat capacity per mole, but as a substance it does not have remarkable heat capacity in terms of volume, mass, or atom-mol (which is just 1.41 R per mole of atoms, or less than half of most solids, in terms of heat capacity per atom). If specific heat is expressed per mole of atoms for these substances, none of the constant-volume values exceed, to any large extent, the theoretical Dulong–Petit limit of 25 J⋅mol −1⋅K −1 = 3 R per mole of atoms (see the last column of this table). Note that the especially high molar values, as for paraffin, gasoline, water and ammonia, result from calculating specific heats in terms of moles of molecules. Generally the most constant parameter is notably the volumetric heat capacity (at least for solids) which is around the value of 3 megajoule per cubic meter per kelvin: ρ c p ≃ 3 MJ / ( m 3 ⋅ K ) (solid) The table of specific heat capacities gives the volumetric heat capacity as well as the specific heat capacity of some substances and engineering materials, and (when applicable) the molar heat capacity. Here's a plot of its heat capacity compared with aluminum and lead.For some substances and engineering materials, includes volumetric and molar values
As a result, its heat capacity is unusually small even for relatively large values of $T$. The rigidity it gets from its somewhat unique crystal structure makes its effective $\omega$ very large. If $T/\hbar\omega$ is sufficiently small, then the heat capacity of the Einstein solid can even drop below $3k/2$, the heat capacity of the classical ideal gas.ĭiamond is special. When the temperature is less than $\hbar\omega$ (which is the spacing between allowed energy levels), there is a dramatic departure from the classical heat capacity of $3k$ because a small parcel of energy changes the temperature quite substantially.
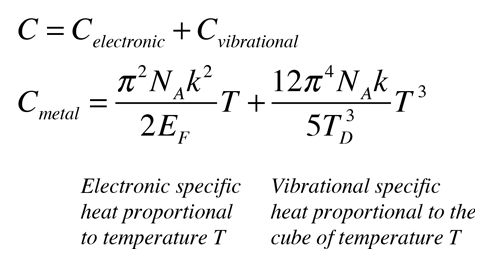
It's the quantum effects that are the key here - specifically the fact that the set of allowed energies for each particle is discrete. $$\fracR$ (note that $R=N_Ak$, where $N_A$ is Avogadro's number) precisely because energy is stored as vibrational potential energy. Temperature is often ( but not always) a measure of the average energy (in the sense that the relationship between temperature and average energy is one-to-one), but in certain systems the relationship is more complicated. This is not a good definition of temperature. Since average KE is literally a definition of temperature


 0 kommentar(er)
0 kommentar(er)
